Any motion of a plane, that maintains its orientation is either a rotation or translation.
Any motion of a plane, that changes its orientation is a glide reflection (or transflection).
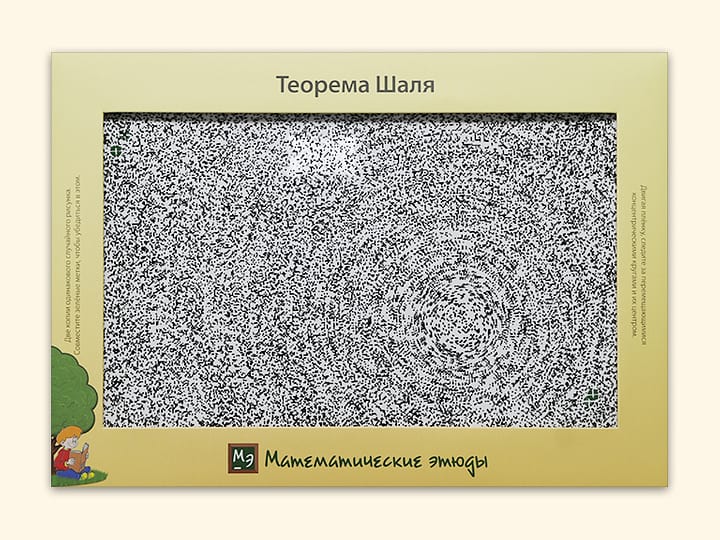
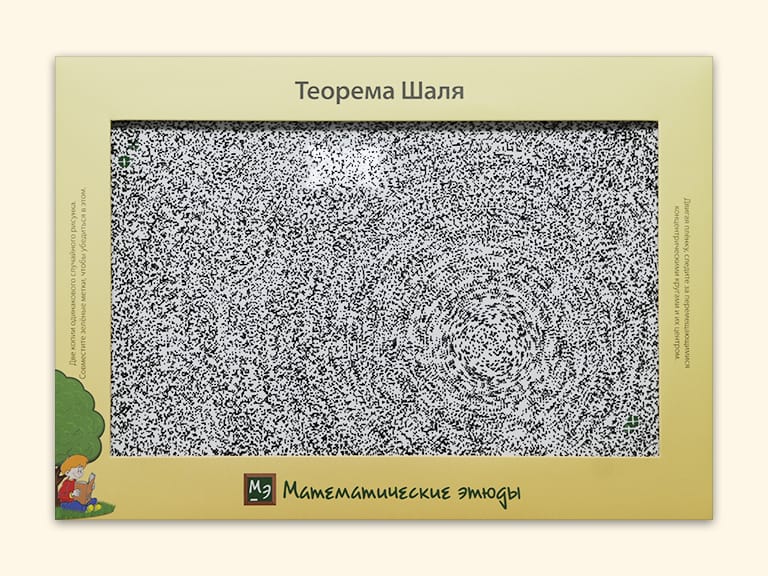
It is possible to make a model, illustrating the first part of this important and interesting geometrical theorem, which has applications, for example, in mechanics. To do this, one needs a sheet of A4 paper, a sheet of transparency film of the same A4 size and a black-and-white printer.
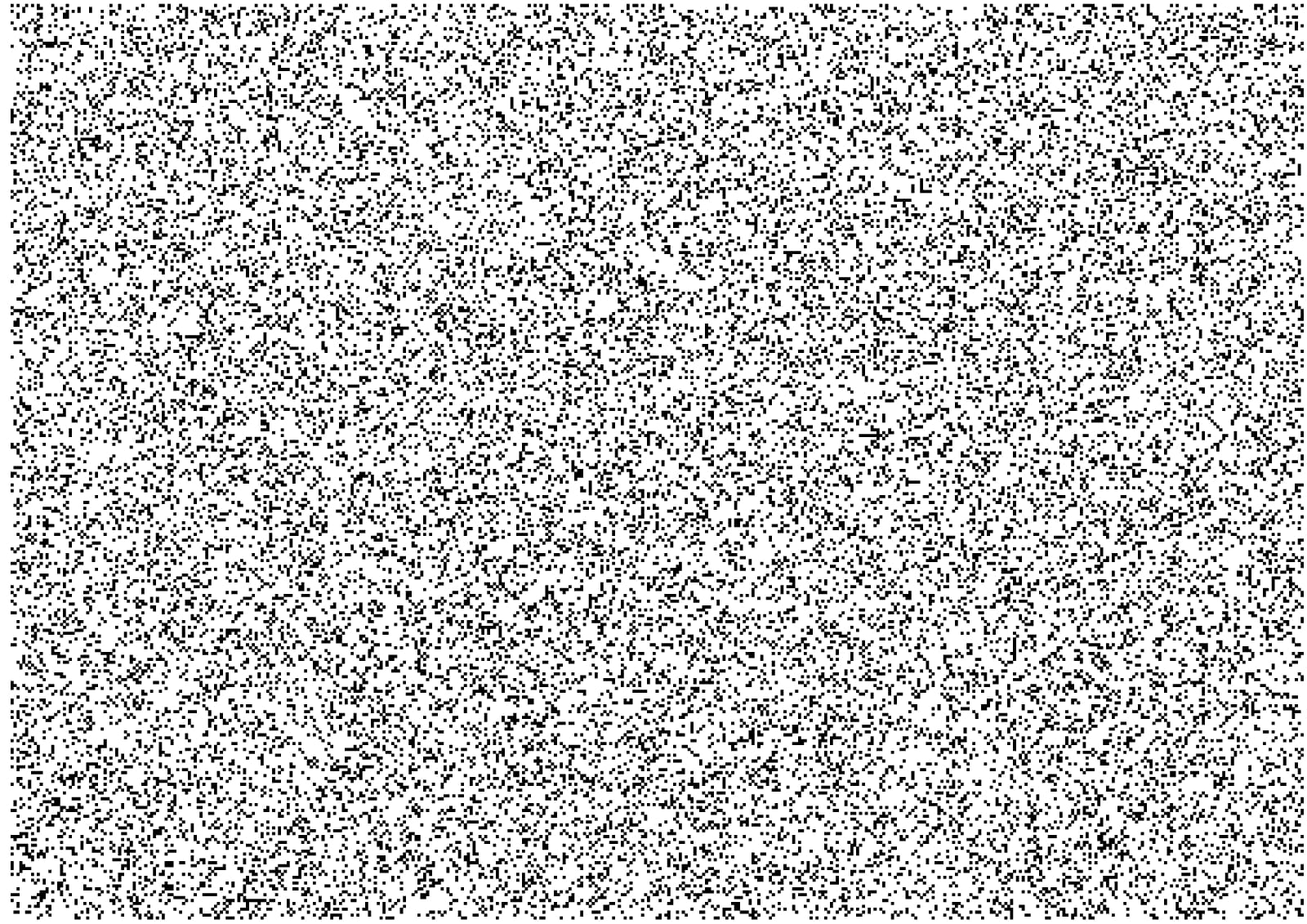
The same drawing — grid with randomly filled cells — is printed on both sheets. Initially, the transparency film is overlaid on the sheet of paper in such a way that the drawings match. Then the film is slightly moved in an arbitrary manner. As a result, the filled cells will almost always be placed on concentric circles and there will be a feeling of seeing these circles (unless one were so unlucky to do a translation). This clearly shows that the motion was a rotation.
The circles are especially prominent when the centre of a rotation falls within the sheet of paper and the rotation angle is not too large. Of course, the theorem is true for any orientation-maintaining film motions relative to a sheet but this example only works for minor translations.
To make such a model, it is just enough to print the same drawing of large number of squares on the sheet of paper and on transparency film. One can create a drawing himself or use the given one. If you are going to do it yourself, be ready to make several attempts: too many or too few squares won’t work well.
You may have seen patterns like this in real life, they are called moirés.